R=a(log2(1+b*c/a*d))
in above equation “a” and “b” are variables.
i write this equation in cvx but could not run.
is this equation ok for above?
-rel_entr((a*d),((a*d)+(b*c)))/log(2)
zaheer
If you tried putting in some “random” numbers for the variables, you would see that is not equivalent to your original formula.
-rel_entr(a,a+b*c/d)/log(2) or -1/d*rel_entr(a*d,a*d+b*c)/log(2) will work.
dear Mark,
if R=B*alpha*log2(1+power*gama0*pathloss/alpha*distance) how i will write it in cvx, is it true or not as
-rel_entr((alpha*distance),((alpha*distance)+(power*gama0*pathloss)))/log(2)??
if not plz suggest me another accurate equation.
moreover, alpha, power are variable in it. plz suggest me. thanks
If you do it that way, you need to divide -rel_entr(...) by distance.
Or instead, use
-rel_entr(alpha,alpha+power*gama0*pathloss/distance)/log(2), which is simpler.
And of course, you need to multiply -rel_entr(....) by B to get R.
When making these reformulations, it is always a good idea to check by using random values (not special values such as 0 or 1) for the inputs to make sure the reformulation matches the original expression to within roundoff error. You should do that to make sure I didn’t make a mistake.
thanks, and B will be multiplied like this;
B(-rel_entr(alpha,alpha+powergama0pathloss/distance)/log(2))?
Yes. Or you can put the B between - and rel_entr so as to avoid use of a pair or parentheses.
humble thanks dear Mark
Of course you need * between B and rel_entr, which I neglected to mention in my previous post.
yes i used it, without * it does not work, thanks
Good morning Mark,
i did this
-St_Data.B*rel_entr(alpha1,alpha1+Temp_N/Temp_D)/log(2)
but got this
Error using * (line 41)
Inner matrix dimensions must agree.
Error in / (line 15)
z = mtimes( x, y, ‘rdivide’ );
whats the problem. even all the terms in above code line are 1x10, only B is scalar.
If Temp_N and Temp_D are both 1 by 10, you need Temp_N./Temp_D
Perhaps you need to brush up on basic MATLAB.
thanks Mark for kind advice
as i did this
Temp_N./Temp_D
this is the result
Error using .* (line 173)
Disciplined convex programming error:
Cannot perform the operation: {real affine} ./ {real affine}
Error in ./ (line 19)
z = times( x, y, ‘./’ );
i did this
times(Temp_N,Temp_D,‘./’)
to resolve error of (line19)
then found this
Error using .* (line 173)
Disciplined convex programming error:
Cannot perform the operation: {real affine} ./ {real affine}
now my question is how to resolve this issue of {real affine} ./ {real affine}.
thanks in advance
dear Mark i don’t know why this error come.
plz suggest me to resolve such issues, before this i also got much help from your comments regarding cvx.
thanks in advance
i have stuck at this point,
even i have checked all possible ways as i know
-1*inv_pos(Temp_D).St_Data.B.rel_entr(alpha1,alpha1+Temp_N)/log(2)
but
Error using . (line 173)
Disciplined convex programming error:
Cannot perform the operation: {concave} . {convex}
i’ll wait for your kind reply.
-St_Data.B*rel_entr((Temp_D),((Temp_D)+(Temp_N)))/log(2)
ans =
cvx concave expression (1x10 vector)
this type give me ok report, where Temp_D, Temp_N are 1x1o matrices while B is scalar.
what do you say it’s enough or i have to divide the whole term with Temp_D?
i show you my original equation to whome i have to code in cvx matlab.
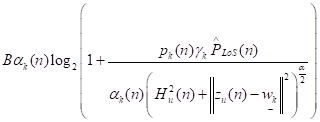
where B is bandwidth, alpha, pk are decision variables, Hu is UAV altitude zu is UAV position, wk is user position. Plos_hat is pathloss which is constant here.
now please suggest me how to code in cvx matlab.
The formulation I provided earlier was valid based on what you said were the variables and constants. When you then showed an expression involving Temp_D, I assumed it was a vector version of the earlier expression, and therefore Temp_D is a constant, which it’s not. Hence the error message.
As for the expression in the most recent post, I have no reason to think it’s either concave or convex, due to the (...)^(alpha/2), which is an exponential. If that expression is concave, you need to prove it.
For your future reference, please note the requirements on the arguments of rel_entr.
help rel_entr
rel_entr Scalar relative entropy.
rel_entr(X,Y) returns an array of the same size as X+Y with the
relative entropy function applied to each element:
{ X.*LOG(X./Y) if X > 0 & Y > 0,
rel_entr(X,Y) = { 0 if X == 0 & Y >= 0,
{ +Inf otherwise.
X and Y must either be the same size, or one must be a scalar. If X and
Y are vectors, then SUM(rel_entr(X,Y)) returns their relative entropy.
If they are PDFs (that is, if X>=0, Y>=0, SUM(X)==1, SUM(Y)==1) then
this is equal to their Kullback-Liebler divergence SUM(KL_DIV(X,Y)).
-SUM(rel_entr(X,1)) returns the entropy of X.
Disciplined convex programming information:
rel_entr(X,Y) is convex in both X and Y, nonmonotonic in X, and
nonincreasing in Y. Thus when used in CVX expressions, X must be
real and affine and Y must be concave. The use of rel_entr(X,Y) in
an objective or constraint will effectively constrain both X and Y
to be nonnegative, hence there is no need to add additional
constraints X >= 0 or Y >= 0 to enforce this.
ooh my dear Mark, i am exhausted now, and jammed.
i’ll check it next morning, what do you say how we will write it in matlab, as i am confused to do something.
thanks for your kind help ever.
i need your help too, for the solution of my data rate equation. if possible please guide me.